Simplify Quadratic Solutions Using Our Complete the Square Calculator
This calculator solves second degree polynomial equations in the form of and quadratic equations using the “complete the square” method.
where a ≠ 0 and ax2 + bx + c = 0.
The answer illustrates the amount of labor needed to complete the square in order to get the real and complex roots of a quadratic equation.
What Does “Completing the Square” Mean?
The quadratic equation in mathematics is solved using the completing the square approach. Using this procedure, the provided equation is transformed so that its left side equals a perfect square binomial. The equation must take the form ax2+ bx+ c=0 in order to be used with this approach. The quadratic formula can be substituted with this method. The quadratic problem that the quadratic formula is unable to solve can be solved by applying the square approach. This is the method to complete the square calculator.
What is the Square’s Completion?
By modifying the left side of the equation to equal the square of a binomial, the approach known as “completing the square” can be used to solve quadratic equations. When factoring is unable to answer the equation, you can apply the complete the square approach.
First, confirm that the term “a” is 1. Divide both sides of the equation by the a term if the result is not 1, and then proceed to finish the square as detailed below.
How to Finish the Square
Completing a quadratic equation’s square requires a few steps.
- To begin, format your equation as follows: ax2 + bx + c = 0.
- Divide both sides of your equation by an if a ≠ 1. After this phase, your terms may be fractional, b and c.
- By deducting or adding to both sides of the equation, move the c term to the right side of the equation.
- Square the result of dividing the b word by two.
- To both sides of the equation, add this outcome.
- Transform the left perfect square into the form (x + y).2.
- Take each side’s square root.
- By deducting or adding the numerical constant on both sides, isolate x on the left.
- Find the value of x. Keep in mind that since you took the square root of the right side of the equation, you will have two solutions: a positive answer and a negative solution.
Finishing the Square in the Case of a Not Equal to One
When an is higher than 1 or less than 1 but not equal to 0, divide both sides of the equation by a to complete the square. This is equivalent to removing all other terms from the value of an.
Let’s finish the square for this quadratic problem as an example:
2×2−12x+7=0
Since a = 2 and a ≠ 1, divide each term by 2.
22×2−122x+72=02 results in
x2−6x+72=0
Utilizing the previously mentioned completing the square method, carry on solving this quadratic equation.
Quadratic Equation
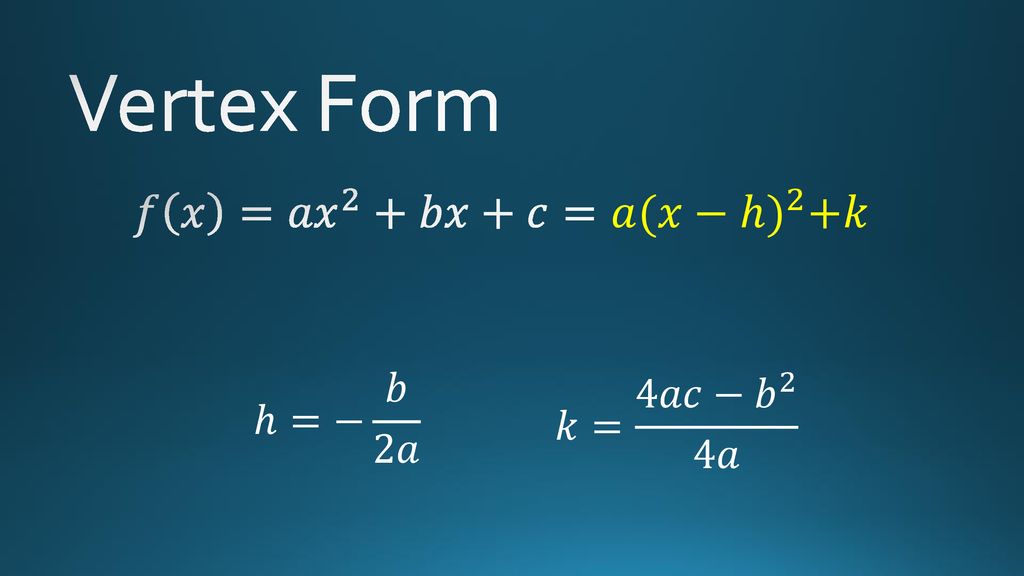
Any polynomial equation of the second degree with the following form is called a quadratic equation in algebra:
ax2 + bx + c = 0
where a, b, and c are the quadratic, linear, and constant coefficients, respectively, and x is an unknown. The coefficients of the equation, denoted by the letters a, b, and c, stand for known numbers. For instance, a cannot equal zero, or else the equation would not be quadratic but rather linear. There are several methods for solving a quadratic equation: factoring, applying the quadratic formula, completing the square, and graphing. Since completing the square is a necessary step in the formula’s derivation, this discussion will only cover the application of the quadratic formula and its fundamentals. The quadratic formula and its derivation are shown below.
Solving Quadratic Equation
Remember that the quadratic equation can have both positive and negative roots when using the ± function to compute the square root. The roots of the quadratic equation, or the x values where any parabola crosses the x-axis, are obtained using the quadratic formula. Moreover, the parabola’s axis of symmetry is likewise given by the quadratic formula. This is seen in the graph that is supplied below. It should be noted that there are numerous practical uses for the quadratic formula, including the computation of areas, projectile trajectories, and speed, among other things. To solve any query, take the help from themathematicsmaster.com.
Conclusion
A function of the form f(x) = ax2 + bx+c, where a, b, and c are real numbers and a does not equal zero, is known as a quadratic function in mathematics. The curve that results from plotting the quadratic function on a graph should resemble a parabola. It is called a “U-Shaped Curve” parabola. Depending on the coefficient sign of “a,” the resulting parabola may face upward or downward, but its width and steepness may vary. A free online tool that shows the quadratic function’s graph is called the Quadratic Function Calculator. The quadratic function calculator tools available on BYJU’s web platform expedite calculations and display the graph in a matter of seconds.